Finding Longitude at Sea

(Note to ID readers: The following is Chapter. XIV of the book I originally wrote for my children and grandchildren – The Life and Times of Matthew Fontaine Maury, Pathfinder of the Seas – offered to you freely and without mental reservation. Keep in mind, as you read, that its contents may serve as a basis for several lessons of study with your own children and/or grandchildren, as well as several related lessons of study, if you so desire. Without further ado.)

Chapter XIV: Finding Longitude at Sea

Were one to draw a series of equally spaced parallel lines on a sheet of paper, then cross them at right angles with another series of parallel lines, also equally spaced on the same sheet of paper, he will have made a grid consisting of a definite number of squares of equal dimensions. Were he to mark a dot at the intersection of any two lines on the grid, and another dot at the intersection of any other two lines on the grid, he could determine at a glance, by counting squares between the two dots, what is the distance, left-to-right and top-to-bottom, between the two dots, or points on the grid. But this is not all.

Were he then to assign a number to each vertical line on the grid as 1,2,3,4,5, etc., and to each horizontal line a letter as, A,B,C,D,E, etc., he may then determine at what position on the grid each of his two dots is at in relation to all other points on the grid. Thus he has a set of coordinates for points on his grid, as D-2, E-4, A-5, and so on. Our draftsman has made a simple Coordinate Grid.

---

Navigators long ago realized that a convenient way for determining a ship’s position at sea relative to known fixed objects, would be by artificially dividing the earth’s surface into coordinates similar to the simple grid in our example above. This system is called Latitude and Longitude, and is a branch of [*] Mathematical Geography. Lines of Latitude run east and west, while lines of Longitude extend north and south through the earth’s poles. Unlike our simple grid, however, lines of latitude and of longitude are assigned numbers of degrees of angular measurement corresponding with their actual positions on a map or a globe.

Points on the line forming a circle are angular measurements called degrees, and there are 360 degrees in any circle regardless of its size or dimensions. Since the earth is a sphere, or circular in shape, a straight line drawn around any part of its surface will form a circle containing 360 degrees of angular measurement.

A right angle is formed by the intersection or crossing of two straight lines extending perpendicular to one another, as on our simple grid described above. If one divided a circle into four equal parts by lines extending outward from the point of the circle’s exact center, he will have formed four right angles. The angular distance in any right angle is ninety degrees. Hence, if we imagine the card of a compass placed about the center of a circle with north being zero degrees, when we move clockwise around the cardinal points of the compass (N.,E.,S.,W.), east will be found to be at ninety degrees from north, south at one hundred eighty degrees from north and ninety degrees from east, west will be at two hundred seventy degrees from north and ninety degrees from south, and north at zero or three hundred sixty degrees to complete our circle and ninety degrees from west.

Circumference is the complete distance around a circle, beginning at any given point on and tracing the circle in one direction, until returning to the point at which we began. The earth is a sphere, or shaped like a ball. It has been measured to be almost 25,000 miles around its surface at its broadest circumference, the equator. The line inscribing the equator divides the earth into two equal halves, northern and southern, called hemispheres, or, half-spheres. If we divide 25,000 mi. by 360 degrees, we get roughly 69 miles. So, the distance from one degree of longitude to the next degree of longitude on the equator equals roughly 69 miles. However, as we move north or south from the equator towards the poles in each hemisphere, the distance between lines of longitude on our grid gradually narrow or come closer together the further north or south our lines extend until they all converge or cross one another at the poles. Distance between degrees of latitude, on the other hand, remain constant from the equator to the poles. This is because, unlike lines of longitude, lines of latitude do not converge at any points opposite one another as do lines of longitude at the north and south poles. Lines of latitude are also and therefore called parallels.

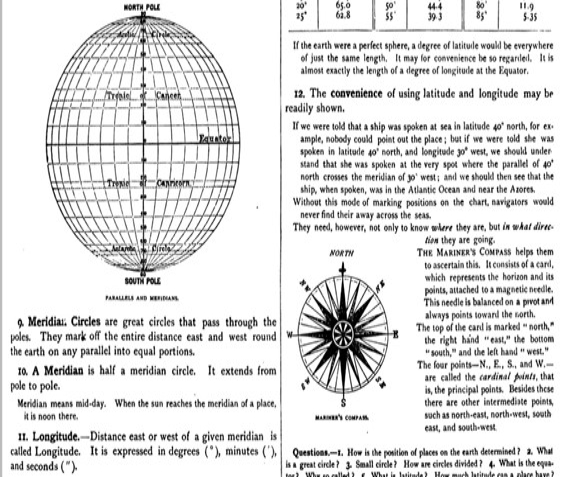
Nevertheless, each degree of latitude and longitude is divided further into smaller increments or fractions of degrees called minutes and seconds. There are sixty minutes in a degree, and sixty seconds in a minute. This division of degrees into the smaller increments of minutes and seconds is useful and needed since, without further dividing degrees into smaller increments, or units of measure, the closest one could place his actual position on the earth would be within a square of 69 miles. Undoubtedly one would know that his position was not at 17 degrees north latitude precisely, but he needs a convenient way to express precise location. Hence, instead of 17 degrees north latitude, minutes and seconds allows the practical navigator to express his ship’s position more precisely, as 17 degrees, three minutes, thirty seconds north. It is easy to convert those angular measurements into miles and parts of miles by way of simple division. If one degree equals sixty-nine miles, and there are sixty minutes in one degree, then he need merely divide 69 by 60 to know that each minute of a degree represents 1.15 miles. Likewise, if he divides 1.15 miles by the number of seconds in one minute (again, 60), we know that each second of a degree is roughly two one hundredths of 1.15 miles.

As a matter of practical navigation, it is generally not necessary to plot a ship’s position more precisely than within the 1.15 miles of a minute on the scale. However, our coordinate grid must be projected into the heavens to be useful to us in plotting positions at sea by use of astronomical observations. And it is in the vast expanse of the heavens where further division of minutes into seconds is the most useful.

If one knows the precise location of a fixed object at sea such as a small island, then he can know where his ship is in relation to the island and therefore how to sail to or around it, as he desires, if he has plotted his position correctly. If he hasn’t plotted his position correctly, then disaster for his ship and all aboard may well be looming on the horizon.

So being able to accurately plot one’s position at sea is very important. But for hundreds of years navigators could only plot their positions at sea semi-accurately. Because their methods yielded results that were imprecise, during any part of a long voyage they could be off by many miles in any conceivable compass direction in their estimations of where they might be. One reason their calculations were very often wrong was because no one had yet discovered a reliable way for finding precise longitude at sea.

The difficulty of finding longitude at sea made seafaring a terror for many sailors and maritime commerce a risky venture because it was the source of many maritime disasters in which entire cargoes were lost or destroyed and whole crews of ships were killed. Navigators could find their latitude at sea easily enough simply by making celestial observations of the sun during the day, or of the North Star at night, when sailing in the northern hemisphere. The North Star is called Polaris. Since Polaris is a “fixed star” situated in the heavens almost directly above the North Pole, one’s latitude is easily determined by measuring the angular distance from the horizon to the star. If the angular distance measured between the horizon and Polaris is 37 degrees, for example, then one’s position is at 37 degrees north latitude. In the northern hemisphere, the angular distance between the horizon and Polaris exactly corresponds with the latitude one is taking the measurement from, so it is very simple to determine latitude at sea when the skies are clear. But longitude cannot be so easily measured by celestial observations, whether the skies are clear or not.

The reason longitude cannot readily be determined by celestial observations is simply because the earth rotates about its axis from west to east. Lines of longitude run north and south, but the angular distances between them are measured east and west. Since the earth rotates from west to east, observations of celestial objects alone cannot tell one where he is longitudinally. This is because a ship’s movement on the sea in a longitudinal direction (due east or due west) does not change the star field or the position of the sun above the horizon. Only changes of latitude and the earth’s ever-changing position on its orbital plane about the sun does that.

Since longitude cannot readily be measured by celestial observations alone, other methods for finding longitude at sea had to be discovered and invented. One way for determining longitude at sea was called dead reckoning. The way it was done was by determining the speed at which a ship was moving on the water, and calculating distance traveled over time based on that speed. The problem was, however, that it was a highly inaccurate method because the speed of a sailing vessel can and often does vary greatly over the course of several hours time. Ship’s captains would, therefore, try to calculate their ship’s average speed during that time, but that was a very imprecise method as well because ships were often times moved along at greater and lesser speeds by currents that were imperceptible to their crews. In short, they were guessing. So maritime nations knew that a better system had to be discovered, and they started to work on solving the longitude problem early in the 18th Century, or, the 1700s.

During that time there was a clock maker in England named John Harrison. Mr. Harrison was a master carpenter by trade and therefore found it convenient to make his clocks of wood. For many years, Mr. Harrison had been making wooden clocks that were self-lubricating and the most accurate timekeepers in England. He knew that the way to solve the longitude problem was to make a clock that would keep perfect, or near perfect time at sea. If a timekeeper could be made that would keep perfect time at sea, he correctly reasoned, then longitude at sea could easily be determined at any time or place on a cruise by setting the sea clock to the exact time at home port upon sailing. In this way all a ship’s captain or master would need to do is mark the time where he was at sea (for example, noon), and compare it to the time on the clock set to keep the time of the home port, or of the port of departure. If the difference between the ship’s time and that at home port were three hours, for example, then the captain would know exactly how far away from port he was longitudinally since he also knew the distance the sun traverses in its apparent motion east-to-west in three hours time.

By that time, mechanical time keepers, as they were called, had been developed that could keep very accurate time on land, but none had yet been made that could keep accurate time at sea. Harrison realized that if he could figure out how to make a timekeeper for use at sea as accurate as those he had been making for use on land, then his “sea clock” would solve the longitude problem that had baffled so many for so long. But how would he do it?

The problem with keeping accurate time at sea was a compound one, meaning that it was several smaller problems that combined to form one big problem. The frequently changing weather conditions at sea was one problem that played havoc on time keepers of the day, but there was also the problem of centrifugal forces when a ship would sway in the water, or when it was in a turn. These forces would disrupt the motions of the pendulums of clocks in those days, causing the clock to either speed up or to slow down, in the same way the same forces cause a man to walk funny to keep his balance on the deck of a rolling or pitching ship. And since a ship is constantly pitching and rolling in the ocean to one degree or another, centrifugal and other forces were likewise constantly disrupting the clock’s ability to operate freely and keep accurate time. Over the course of a long voyage a typical clock might lose many minutes or even hours of time, making the clock useless for determining longitude at sea. And no one knew how to prevent this from happening.

Undeterred by what seemed like the impossibility of solving the problem facing him, Mr. Harrison went to work on finding a solution to making such a clock. He conceived a system of counter-balances and springs that he thought would compensate for the rolling and pitching motions of a ship at sea. This was an improvement over any design that had been tried before, but his clock still lost or gained too much time over long voyages when put to the test. At first he couldn’t understand why, but by persistence he finally began to realize that centrifugal force was the culprit. So he began to build another clock with different kinds of balances that he thought would compensate for both forces. But in the middle of the project he realized that his new design of the balances still wouldn’t produce the results needed for reliably determining longitude at sea. He also realized that his clocks were too big and unwieldy to be of practical use on ships at sea. Nevertheless, his dedicated work and persistence of many years finally paid off.

After more than forty years of unceasing labor, conceiving of and trying new designs and ideas that failed many times over, Mr. Harrison was able to conceive of a radically different fourth design which was much smaller and more compact than his earlier attempts, and that would not be affected by centrifugal and centripetal forces caused by a ship’s pitching and rolling, nor its yawing and turning motions at sea. Mr. Harrison finally succeeded in designing and building a timepiece that could be relied upon to keep accurate time at sea. This timepiece, or “sea clock,” was called the Marine Chronometer, and must of itself and according to the will of Almighty God, be responsible for having lengthened many a seaman’s life, and of saving untold millions in the value of ships and cargoes lost had this indispensable timepiece not been invented and made; this timepiece was instrumental in Commander Maury’s later work of discovering and plotting the paths of the seas, as spoken of in Psalm 8.

---

[*] https://identitydixie.com/2020/09/22/virtual-schooling-and-maury-map-drawing/